SOCR EduMaterials Activities Poisson Distribution

This is an activity to explore the Poisson Probability Distribution.

• Description: You can access the applets for the above distributions at http://www.socr.ucla.edu/htmls/SOCR_Distributions.html .

• Exercise 1: Use SOCR to graph and print the distribution of a Poisson random variable with \( \lambda=2 \). What is the shape of this distribution?

• Exercise 2: Use SOCR to graph and print the distribution of a Poisson random variable with \( \lambda=15 \). What is the shape of this distribution? What happens when you keep increasing \( \lambda \)?

• Exercise 3: Let \( X \sim Poisson(5) \). Find \( P(3 \le X < 10) \), and \( P(X >10 | X \ge 4) \).

• Exercise 4: Poisson approximation to binomial: Graph and print \( X \sim b(60, 0.02) \). Approximate this probability distribution using Poisson. Choose three values of \( X \) and compute the probability for each one using Poisson and then using binomial. How good is the approximation?

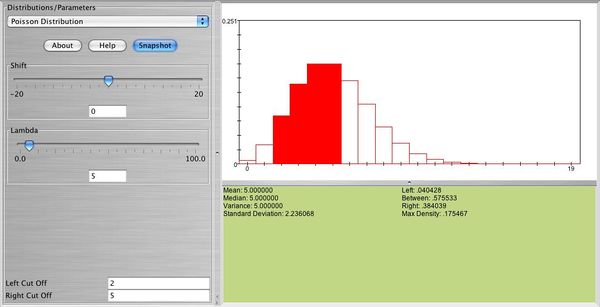
Below you can see the distribution of a Poisson random variable with \( \lambda=5 \). In this graph you can also see the probability that between 2 and 5 events will occur.

• Exercise 5: People enter a gambling casino at a rate of 1 for every two minutes.
  • What is the probability that no one enters between 12:00 and 12:05?
  • What is the probability that at least 4 people enter the casino during that time?

• Exercise 6: Let \(X_1\) denote the number of vehicles passing a particular point on the eastbound lane of a highway in 1 hour. Suppose that the Poisson distribution with mean \(\lambda_1=5\) is a reasonable model for \(X_1\). Now, let \(X_2\) denote the number of vehicles passing a point on the westbound lane of the same highway in 1 hour. Suppose that \(X_2\) has a Poisson distribution with mean \(\lambda_2= 3\). Of interest is \(Y= X_1 + X_2\), the total traffic count in both lanes in one hour. What is the \(P(Y < 5) ?\)

---

• SOCR Home page: http://www.socr.ucla.edu

---

---

Translate this page:

(default)	Deutsch	Español	Français	Italiano	Português	日本語	България	الامارات العربية المتحدة	Suomi	इस भाषा में	Norge
한국어	中文	繁体中文	Русский	Nederlands	Ελληνικά	Hrvatska	Česká republika	Danmark	Polska	România	Sverige

</math>