SOCR EduMaterials Activities ApplicationsActivities BinomialOptionPricing

SOCR Applications Activities - Binomial Option Pricing Model

Description

You can access the Binomial Option Pricing Model applet at the SOCR Applications Site, select Financial Applications --> BinomialOptionPricing.

Definitions

$$S_0$$ Stock price at $t=0$
$$S_1$$ Stock price at $t=1$
$$E$$ Exercise price of the call option
$$u$$ $1+ \%$ change in stock price from $$t=0$$ to $t=1$ if stock price increases ($u>1$) $$d$$ $1+ \%$ change in stock price from $$t=0$$ to $t=1$ if stock price decreases ($d<1$) $$C$$ The call price
$$\alpha$$ The number of shares of stocks purchased per one call (hedge ratio)
$$C_u$$ Price of call at $t=1$ if stock price increases $$max(S_1-E,0)$$ or $max(uS_0-E,0)$
$$C_d$$ Price of call at $t=1$ if stock price decreases: $$max(S_1-E,0)$$ or $max(dS_0-E,0)$
$$r$$ Continuous risk-free interest rate

• The value $C$ of a European call option at time $t=0$ is:

$$C=S_0 \sum_{j=k}^{n} {n \choose j} p'^{j}(1-p')^{n-j} - \frac{E}{e^{rt}} \sum_{j=k}^{n} {n \choose j} p^{j}(1-p)^{n-j}$$
Where,
$$u=e^{+\sigma \sqrt{\frac{t}{n}}}, d=e^{-\sigma \sqrt{\frac{t}{n}}}=\frac{1}{u}$$
$$p=\frac{e^{rt}-d}{u-d}, \ \ p'=\frac{up}{e^{rt}}$$

$$S_0$$ Price of the stock at time $t=0$
$$E$$ Exercise price at expiration
$$r$$ Risk-free interest rate per period
$$n$$ Number of periods
$$\sigma$$ Annual standard deviation of the returns of the stock
$$t$$ &Time to expiration in years

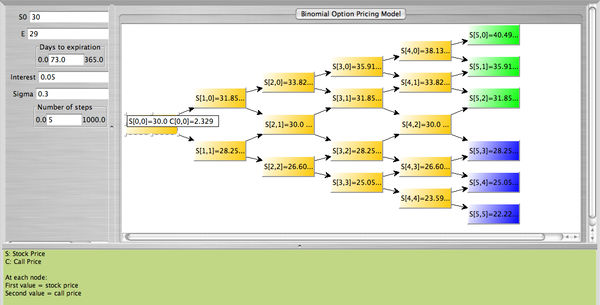
• The SOCR Binomial Option Pricing applet provides the price of the stock and the price of the call at each node. Note that at expiration the nodes for which the call is in the money ($S > E$ are colored green, while the nodes for which the call is out of the money ($S \le E)$ are colored blue. The example below uses the following data:
  

$$S_0=\$30$$ , $E=\$29$, $r_f=0.05$, $\sigma=0.30$, $\mbox{days to expiration}=73$, $\mbox{number of steps}=5$.

References

The materials above was partially taken from:

• Modern Portfolio Theory by Edwin J. Elton, Martin J. Gruber, Stephen J. Brown, and William N. Goetzmann, Sixth Edition, Wiley, 2003.
• Options, Futues, and Other Derivatives by John C. Hull, Sixth Edition, Pearson Prentice Hall, 2006.

• SOCR Applications Site

---

---

Translate this page:

(default)	Deutsch	Español	Français	Italiano	Português	日本語	България	الامارات العربية المتحدة	Suomi	इस भाषा में	Norge
한국어	中文	繁体中文	Русский	Nederlands	Ελληνικά	Hrvatska	Česká republika	Danmark	Polska	România	Sverige