Difference between revisions of "AP Statistics Curriculum 2007 Cauchy"
m (Text replacement - "{{translate|pageName=http://wiki.stat.ucla.edu/socr/" to ""{{translate|pageName=http://wiki.socr.umich.edu/") |
|||
| (3 intermediate revisions by one other user not shown) | |||
| Line 2: | Line 2: | ||
=== Cauchy Distribution === | === Cauchy Distribution === | ||
| − | The standard Cauchy distribution is derived from the ratio of two independent Normal | + | The standard Cauchy distribution is derived from the ratio of two independent [http://wiki.stat.ucla.edu/socr/index.php/AP_Statistics_Curriculum_2007_Normal_Std Normal Distributions]. If X ~ N(0,1), and Y ~ N(0,1), then <math>\tfrac{X}{Y} \sim Cauchy(0,1)</math> |
The Cauchy distribution is important in physics (where it’s known as the Lorentz distribution) because it’s the solution to the differential equation describing forced resonance. In spectroscopy, it is the description of the shape of spectral lines which are subject to homogeneous broadening in which all atoms interact in the same way with the frequency range contained in the line shape. | The Cauchy distribution is important in physics (where it’s known as the Lorentz distribution) because it’s the solution to the differential equation describing forced resonance. In spectroscopy, it is the description of the shape of spectral lines which are subject to homogeneous broadening in which all atoms interact in the same way with the frequency range contained in the line shape. | ||
| Line 16: | Line 16: | ||
'''Median''': <br> | '''Median''': <br> | ||
| + | <math>x_0\!</math> | ||
| + | |||
| + | '''Mode''': <br> | ||
<math>x_0\!</math> | <math>x_0\!</math> | ||
| Line 24: | Line 27: | ||
<math>x \in (-\infty, +\infty)\!</math> | <math>x \in (-\infty, +\infty)\!</math> | ||
| − | '''Moment Generating Function''' | + | '''Moment Generating Function''' <br> |
Does Not Exist | Does Not Exist | ||
| Line 49: | Line 52: | ||
* SOCR Home page: http://www.socr.ucla.edu | * SOCR Home page: http://www.socr.ucla.edu | ||
| − | {{translate|pageName=http://wiki. | + | "{{translate|pageName=http://wiki.socr.umich.edu/index.php/AP_Statistics_Curriculum_2007_Cauchy}} |
Latest revision as of 13:15, 3 March 2020
Contents
General Advance-Placement (AP) Statistics Curriculum - Cauchy Distribution
Cauchy Distribution
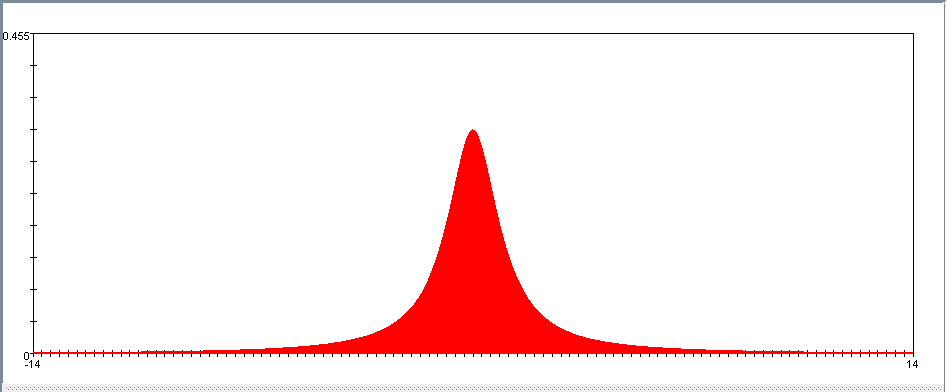
The standard Cauchy distribution is derived from the ratio of two independent Normal Distributions. If X ~ N(0,1), and Y ~ N(0,1), then \(\tfrac{X}{Y} \sim Cauchy(0,1)\)
The Cauchy distribution is important in physics (where it’s known as the Lorentz distribution) because it’s the solution to the differential equation describing forced resonance. In spectroscopy, it is the description of the shape of spectral lines which are subject to homogeneous broadening in which all atoms interact in the same way with the frequency range contained in the line shape.
PDF:
\(\frac{1}{\pi\gamma\,\left[1 + \left(\frac{x-x_0}{\gamma}\right)^2\right]}\!\)
CDF:
\(\frac{1}{\pi} \arctan\left(\frac{x-x_0}{\gamma}\right)+\frac{1}{2}\!\)
Mean:
Does Not Exist
Median:
\(x_0\!\)
Mode:
\(x_0\!\)
Variance:
Does Not Exist
Support:
\(x \in (-\infty, +\infty)\!\)
Moment Generating Function
Does Not Exist
Applications
\(\cdot\) Used in mechanical and electrical theory, physical anthropology and measurement and calibration problems.
\(\cdot\) In physics it is called a Lorentzian distribution, where it is the distribution of the energy of an unstable state in quantum mechanics.
\(\cdot\) Also used to model the points of impact of a fixed straight line of particles emitted from a point source.
Example
SOCR Links
http://www.distributome.org/ -> SOCR -> Distributions -> Distributome
http://www.distributome.org/ -> SOCR -> Distributions -> Cauchy Distribution
http://www.distributome.org/ -> SOCR -> Functors -> Cauchy Distribution
SOCR Cauchy Distribution calculator (http://socr.ucla.edu/htmls/dist/Cauchy_Distribution.html)
- SOCR Home page: http://www.socr.ucla.edu
"-----
Translate this page: