UQuadraticDistribuionAbout

About_pages_for_SOCR_Distributions - U-Quadratic Distribution

Description

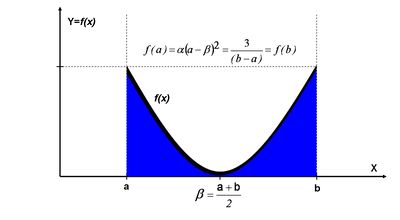
The U quadratic distribution is defined by the following density function

\( f(x)=\alpha \left ( x - \beta \right )^2, \forall x \in [a , b], a < b\),

where the relation between the two pairs of parameters (domain-support, a and b) and (range/offset \(\alpha\) and \(\beta\)) are given by the following two equations

(gravitational balance center) \(\beta = {b+a \over 2}\), and (vertical scale) \(\alpha = {12 \over \left ( b-a \right )^3}\).

More information about U-quadratic, and other continuous distribution functions, is available at Wikipedia.

Properties

• Support Parameters\[a < b \in (-\infty,\infty)\]
• Scale/Offset Parameters\[\alpha \in (0,\infty)\] and \(\beta \in (-\infty,\infty)\)
• PDF\[f(x)=\alpha \left ( x - \beta \right )^2, \forall x \in [a , b]\]
• CDF \(F(x)={\alpha \over 3} \left ( (x - \beta)^3 + (\beta - a)^3 \right ), \forall x \in [a , b]\)
• Mean\[{a+b \over 2}\]
• Median\[{a+b \over 2}\]
• Modes\[a \] and \( b \)
• Variance\[ {3 \over 20} (b-a)^2 \]
• Skewness: 0 (distribution is symmetric around the mean)
• Kurtosis\[ {3 \over 112} (b-a)^4 \]
• Moment Generating Function\[M_x(t)= {-3\left(e^{at}(4+(a^2+2a(-2+b)+b^2)t)- e^{bt} (4 + (-4b + (a+b)^2)t)\right) \over (a-b)^3 t^2 }\]
• Characteristic Function\[{3i\left(e^{iat}(-4i+(a^2+2a(-2+b)+b^2)t)+ e^{ibt} (4i - (-4b + (a+b)^2)t)\right) \over (a-b)^3 t^2 }\]

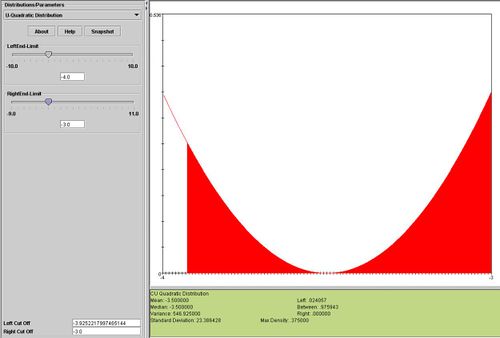
Interactive U Quadratic Distribution

You can see the interactive U Quadratic distribution by going to SOCR Distributions and selecting from the drop down list of distributions U Quadratic. Then follow the Help instructions to dynamically set parameters, compute critical and probability values using the mouse and keyboard.

---

• SOCR Home page: http://www.socr.ucla.edu
• U-quadratic distribution at Wikipedia

---

---

Translate this page:

(default)	Deutsch	Español	Français	Italiano	Português	日本語	България	الامارات العربية المتحدة	Suomi	इस भाषा में	Norge
한국어	中文	繁体中文	Русский	Nederlands	Ελληνικά	Hrvatska	Česká republika	Danmark	Polska	România	Sverige