SOCR APS Apr 2023 ILT Shen
Contents
SOCR News & Events: Numerical Methods and Analysis for Computing Forward and Inverse Laplace Transform For discrete and continuous signals
Presenter
- Yueyang Shen is a PhD candidate in the Department of Computational Medicine and Bioinformatics and enrolled as Trainee in the Biomedical Informatics and Data Science Program at the University of Michigan.
Session Logistics
- Session: WW03: V: General Physics III; APS April Meeting 2023, Minneapolis, Minnesota (Apr 15-18), Virtual (Apr 24-26); Time Zone: Central Time
- Chair: Alexey Grinin, Northwestern University, Center for Fundamental Physics, Room: Virtual Room 3
Abstract
- Specialized data maps transforming (probabilistic) state representations mapping the original signal representation into a more manipulatable state space capitalizes on contemporary computational advances and relates to well-known application scenarios, such as kernel machines, MDP transition dynamics modeling and policy optimization, and generative modeling. In this work we focus on the classic example of Laplace transform, which maps the subspace of differential equations to the space of algebraic equations, where the differential equation solution can be obtained by inverting the algebraic equation solution. Effective integral transformations enable simplification of mathematical modeling and computational inference. The Laplace transform (LT) with its inverse (ILT) represents a family of integral transforms that have direct applications in contemporary data science, statistical inference, and probabilistic modeling. However, practical computational challenges inhibit their utilization on complex or implicit functions, noisy observations, and incomplete data.
- We propose a numerical LT-ILT computing framework, empirically robust, implemented in R, and facilitates numerical computations through appropriate parameter estimations and signal approximations. We illustrate an idealized analysis relaxing the data matrix construction assumptions to bound the smallest singular values to argue algorithmic stability according to theoretical results in random matrix theory.
Paper & Slidedeck
- Paper: DOI: https://doi.org/10.48550/arXiv.2304.13204
- Slides
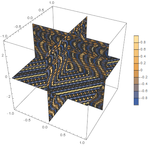
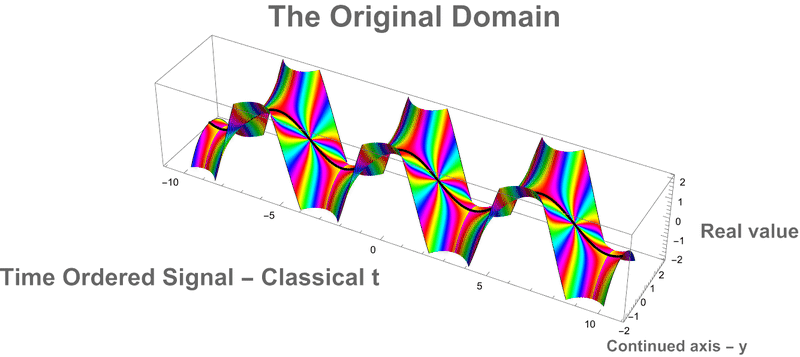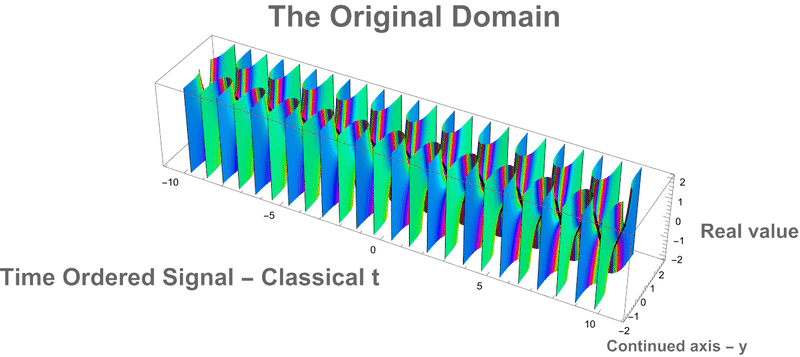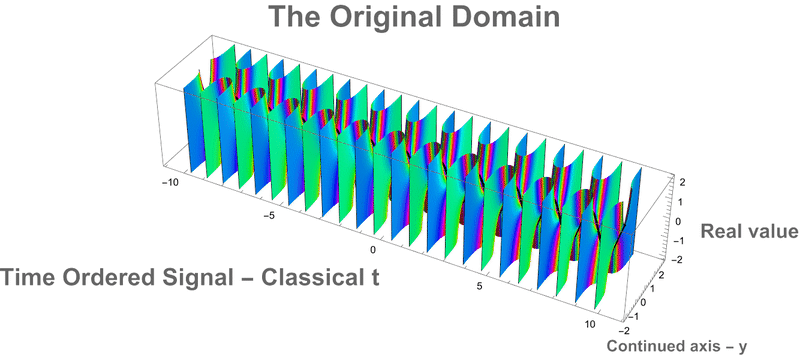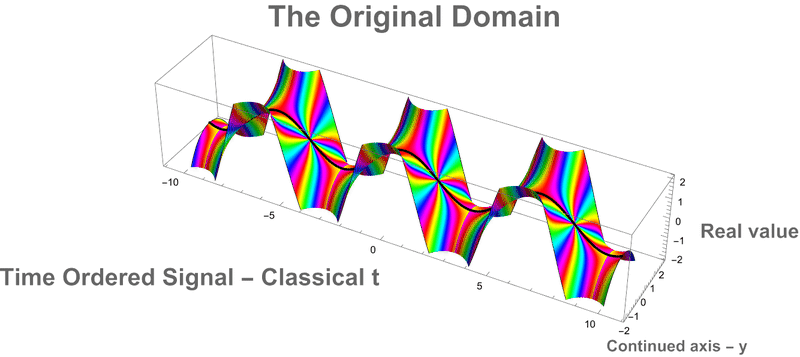
Example: Time-series to Kime-surface Mapping
The first animation shows a spatially-extended time-series wave, whereas the second animation illustrates the corresponding kime-surface obtained via the Laplace transform (LT). More details are available on the TCIU/Spacekime analytics website.
Background
- SOCR News & Events
- SOCR Global Users
- SOCR Navigators
- SOCR Datasets and Challenging Case-studies
- Electronic Textbooks:
References
- Dinov, ID and Velev, MV (2021) Data Science: Time Complexity, Inferential Uncertainty, and Spacekime Analytics, De Gruyter (STEM Series), Berlin/Boston, ISBN 9783110697803 / 3110697807, DOI 10.1515/9783110697827.
- Wang, Y, Shen Y, Deng, D, Dinov, ID. (2022) Determinism, Well-posedness, and Applications of the Ultrahyperbolic Wave Equation in Spacekime, Journal of Partial Differential Equations in Applied Mathematics, DOI: 10.1016/j.padiff.2022.100280, in press.
- Zhang, R, Zhang, Y, Liu, Y, Guo, Y, Shen, Y, Deng, D, Qiu, Y, Dinov, ID. (2022) Kimesurface Representation and Tensor Linear Modeling of Longitudinal Data, Neural Computing and Applications Journal, DOI: 10.1007/s00521-021-06789-8.
- Spacekime website
- Time-Complexity and Inferential Uncertainty (TCIU)
Translate this page: