SOCR EduMaterials Activities PokerDiceeriment
Poker Dice Experiment
Description
The poker dice experiment consists of rolling 5 fair dice. Random variable V denotes the value of the hands
V = 1: one pair, V = 2: two pair, V = 3: three of a kind, V = 4: full house, V = 5: four of a kind, V = 6: five of a kind.
The die scores and the value V are recorded on each update in the first table. The density function of V is shown blue in the graph are recorded in the second table. On each update, the empirical density function of V is shown in red in the red graph and recorded in the second table. In the stop frequency list box, you can set the simulation to stop automatically when V is a particular value.
Goal
To provide a simulation demonstrating a different type of conditional probability involving five fair dices and the value of hands, and to give a generalization of more complicated probability events. After applying this applet to other kinds of events, users should be able to develop a better understanding regarding outcomes and characteristics of events.
Experiment
Go to the SOCR Experiment [[1]] and select the Poker Dice Experiment from the drop-down list of experiments on the top left. The image below shows the initial view of this experiment:
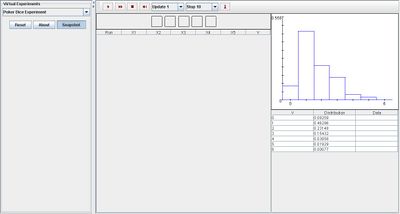
When pressing the play button, one trial will be executed and recorded in the distribution table below. The fast forward button symbolizes the nth number of trials to be executed each time. The stop button ceases any activity and is helpful when the experimenter chooses “continuous,” indicating an infinite number of events. The fourth button will reset the entire experiment, deleting all previous information and data collected.
The “update” scroll indicates nth number of trials (1, 10, 100, or 1000) performed when selecting the fast forward button and the “stop” scroll indicates the maximum number of trials in the experiment.
AS the number of trials increase, the empirical density graph converges to the distribution graph:
Applications
The Poker Dice Experiment is an example demonstrating the properties of implementing probabilities with or without conditions. For example,
Suppose a dealer wants to be able to demonstrate the properties when dealing cards at the casino he works at so he uses this applet for illustration.
Suppose a new gambler wants to determine the effects of probabilities involving poker dice games.
Translate this page: